This post describes the construction of quasianalytic algebras of functions with simple logarithmic transseries as asymptotic expansions. (It is a slightly altered version of this post.)
The resulting algebra of germs contains Ilyashenko’s set $\A$ of almost regular germs introduced previously. The set $\A$ is closed under composition, but it is not closed under addition or multiplication; to obtain a ring, we need to allow finite iterates of the logarithm in the asymptotic expansions.
As in my previous post, I will work in the logarithmic chart at $+\infty$; in particular, composition of germs at $0^+$ becomes log-composition of germs at $+\infty$.
The construction of this algebra is done by iterating Ilyashenko’s construction of $\A$. Among other things, this leads to dealing with asymptotic series that have order type larger than $\omega$, so we first need to define what we mean by “asymptotic expansion”.
Let $G$ be a multiplicative subgroup of some Hardy field of $C^\infty$-germs at $+\infty$, and let $\Gs{\RR}{G}$ denote the corresponding generalized series field. (The support of such a series is a reverse well-ordered subset of $G$.) Assume that $G$ is a scale at $+\infty$, that is, that $G$ is linearly ordered by the dominance relation $\prec$ on real germs at $+\infty$.
Example
Let $L$ be the multiplicative real vector space of germs of the form $\log_{-1}^{\alpha_{-1}} \log_0^{\alpha_0} \cdots \log_k^{\alpha_k}$, where $k \in {-1} \cup \NN$, $\alpha = (\alpha_{-1}, \dots, \alpha_k) \in \RR^{2+k}$ and $\log_i$ denotes the $i$th compositional iterate of $\log$ (so that $\log_0 = x$ and $\log_{-1} = \exp$). Then, by basic calculus, $L$ is a scale at $+\infty$.
Let $K$ be an $\RR$-algebra of $C^\infty$-germs at $+\infty$, and let $T:K \into \Gs{\RR}{G}$ be an $\RR$-algebra homomorphism.
For $F \in \Gs{\RR}G$ and $g \in G$, we denote by $F_g$ the truncation of $F$ above $g$.
Definition
We say that $(K,G,T)$ is a quasianalytic asymptotic (or qaa for short) algebra if
- $T$ is injective;
- $T(K)$ is truncation closed;
- for every $f \in K$ and every $g \in G$, we have
$$
\left| f(x) – T^{-1}((Tf)_g)(x) \right| = o(g(x))
\quad\text{as } x \to +\infty,
$$ or equivalently that $$f – T^{-1}((Tf)_g) \prec g.$$
Our aim is to construct a qaa field $(\K,L,T)$ such that $\K$ contains $\A \circ e^{-z}$. The construction is based on the Uniqueness Principle (UP) discussed in my previous post. To use this principle, we need to work with monomials that have holomorphic continuations to standard quadratic domains:
Definition
We say that $G$ is a scale on $\Omega$, where $\Omega \subseteq \CC$ is a domain such that $\re(z)$ is unbounded above as $z$ ranges over $\Omega$, if every germ $g$ in $G$ has a holomorphic continuation $\bar g$ on $\Omega$, and if for any two distinct $g,h \in G$, we have $$\frac gh(z) \text{ is bounded on } \partial\Omega \text{ and } g(z) = o(h(z))$$ or $$\frac hg(z) \text{ is bounded on } \partial\Omega \text{ and } h(z) = o(g(z))$$ as $|z| \to +\infty$ in $\Omega$.
Examples
- The group $L$ is a scale on every standard quadratic domain. However, $L$ is not a scale on the right half-planes, as the monomial $e^{-z}$ has constant nonzero modulus on any vertical line in $\CC_+$.
- The multiplicative real vector space generated by $L$ and $e^{x^2}$ is not a scale on any standard quadratic domain, nor on any sector of opening at least $\frac\pi4$; but it is a scale on any sector of opening strictly less than $\frac\pi4$.
Thus, to work with $L$ as monomials, we have to work on complex domains that are “smaller” than half-planes, such as standard quadratic domains.
On the other hand, proper subsectors of $\CC^+$ of opening less than $\frac\pi2$ are too small for us, because UP does not apply there anymore (the functions $e^{x^\beta}$ for $\beta < 1$ sufficiently close to 1 are counterexamples to UP on such sectors).
Thus, standard quadratic domains are examples of domains on which both $L$ is a scale and UP holds.
The construction
In view of UP, we define $\A_0$ to be the set of all germs at $+\infty$ of functions $f:\RR \into \RR$ that have a bounded, holomorphic continuation $\f:\Omega \into \CC$ to (the closure of) some standard quadratic domain $\Omega$ and for which there exist real numbers $0 \le \nu_0 < \nu_1 < \cdots$ and $a_0, a_1, \dots$ such that $\lim_{n \to \infty} \nu_n = +\infty$ and $$ \f(z) – \sum_{n=0}^N a_n e^{-\nu_n z} = o\left(e^{-N\re(z)}\right) \quad\text{as}\quad \re(z) \to \infty \text{ in } \Omega, \quad\text{for all } N \in \NN. $$ In this situation, we set $T^0_0f:= \sum_{n=0}^\infty a_n e^{-nx} \in \TT$.
It is easy to see that $\A_0$ is an algebra; by UP, the triple $\left(\A_0, L,T^0_0\right)$ is a qaa algebra.
Remark
The algebra $\A_0 \circ (-\log)$ is the algebra $\A_1$ considered in this paper.
Next, we let $\F_0$ be the fraction field of $\A_0$ and extend $T_0$ to $\F_0$ in the obvious way. Note that the functions in $\F_0$ do not all have bounded holomorphic continuations to standard quadratic domains; hence the need for first defining $\A_0$.
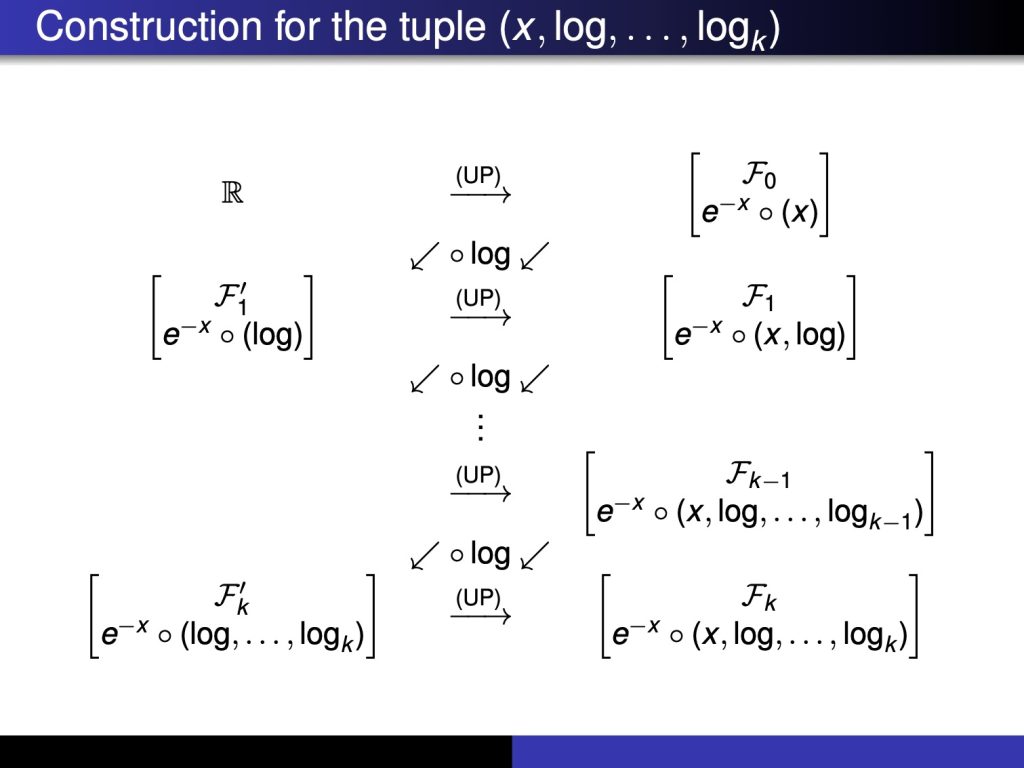
We now construct qaa fields $\left(\F_k, L, T_k\right)$, for $k \in \NN$, such that $\F_k$ is a subfield of $\F_{k+1}$ and $T_{k+1}$ extends $T_k$, as follows: assuming $\left(\F_k,L, T_k\right)$ has been constructed, we set
$$
\F’_{k+1} := \F_k \circ \log
$$
and define $T’_{k+1}:\F’_{k+1} \into \TT$ by $$T’_{k+1}(f \circ \log) := \left(T_k f\right) \circ \log.$$
Since the image of any standard quadratic domain under $\log$ is contained (as a germ at $+\infty$) in every standard quadratic domain, every $f \in \F’_{k+1}$ has a holomorphic continuation $\f:\Omega \into \CC$ on some standard quadratic domain $\Omega$ depending on $f$.
Then $\left(\F’_{k+1},L,T’_{k+1}\right)$ is a qaa field. Moreover, since the series $T’_{k+1}(f)$ for $f \in \F’_{k+1}$ only involve $\log_i$ with $i \ge 0$, the germs in $\F’_{k+1}$ are polynomially bounded at $+\infty$.
We now let $\A_{k+1}$ be the set of all germs at $+\infty$ of functions $f:\RR \into \RR$ that have a bounded, holomorphic continuation $\f:\Omega \into \CC$ to some standard quadratic domain $\Omega$, and for which there exist real numbers $0 \le \nu_0 < \nu_1 < \cdots$ and germs $a_0, a_1, \dots$ in $\F’_{k+1}$ such that $\lim_{n \to \infty} \nu_n = +\infty$ and $$ \f(z) – \sum_{n=0}^N \a_n(z) e^{-\nu_n z} = o\left(e^{-\nu_Nz}\right) \quad\text{as}\quad |z| \to \infty \text{ in } \Omega, \quad\text{for all } N \in \NN. $$ This makes sense, because the $\a_n$ are polynomially bounded at $+\infty$.
In this situation, we set $T_{k+1} f:= \sum_{n=0}^\infty \left(T’_{k+1} a_n\right) \cdot e^{-nx} \in \TT$. By UP and the inductive assumption that $T_{k+1}$ is injective, the triple $\left(\A_{k+1}, L,T_{k+1}\right)$ is again a qaa algebra.
Finally, we let $\F_{k+1}$ be the fraction field of $\A_{k+1}$ and extend $T_{k+1}$ correspondingly.
Remarks
- $\A_1 \circ (-\log)$ contains the set $\A \circ e^{-z}$.
- One shows, by induction on $k$, that both $\F_k$ and $\F’_{k+1}$ are subalgebras of $\F_{k+1}$, and that the restrictions of $T_{k+1}$ to $\F_k$ and $\F’_{k+1}$ are $T_k$ and $T’_{k+1}$, respectively.
In view of Remark 2 above, we set $\F:= \bigcup_k \F_k$ and $T$ be the unique common extension of all $T_k$ to $\F$. It follows that $(\F,L,T)$ is a qaa field.
Note that, by construction and Remark 2, we have $\F \circ \log \subseteq \F$.
Moreover, we get the following:
Theorem
The field $\F$ is a Hardy field closed under log-composition, and $T$ respects both differentiation and log-composition.
For later reference, let me summarize the above construction as follows: for $k \in \NN$, let $L_k$ be the multiplicative real vector space generated by the tuple $$e^{-x} \circ (x, \log, \dots, \log_k) := \left( e^{-x}, x, \log x, \dots, \log_{k-1} x\right);$$ note that $L = \bigcup_{k=-1}^\infty L_k$. Then we construct qaa fields $(\F_k,L_k,T_k)$ according to the following scheme: